[EL / ET] Resistors Association

Reading time: less than 1 minute

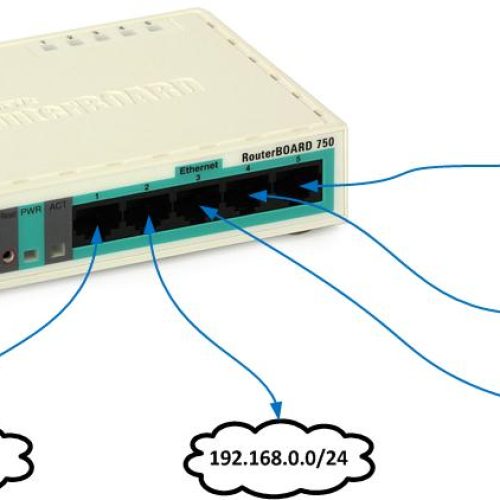
In practical work, is often we need a resistor whose electric resistance value do not have the time, or that is not manufactured by specialized firms. In these cases, solution of the problem is obtained by combining other resistors in order to obtain the desired resistor.
We can associate the most varied forms resistors, but we will give a special mention, in this chapter, the series associations, parallel and mista.
It is important to note that, whatever combination performed, we are always interested in getting the equivalent resistor , that is, obtaining a single resistor, placed between the same points A and B of an association, be subject to the same DDP and is traversed by a current intensity equal to the association.

In electrical circuits uses the concept of it, which is the junction of three or more circuit branches.

Example:

• Are we:

• Are not we:

This concept is very important in the study of associations in series and parallel to an electrical circuit elements.

• Association Series

A set of any said resistors is associated in series When all resistors are traversed by same electric current.
In order to have a series in association, it is necessary that the resistors are connected one after the other, that is, there can be no node between the resistors. The figure below shows an association in series of n resistors.

To determine the resistor equivalent to an association in series of n resistors, we must remember that the electric current is the same, both the resistor equivalent as for resistors associated, and that the equivalent resistor DDP is the sum of each resistor associated DDPS.

• resistor Equivalent

being:

and being

we have:

that is:

The resistor equivalent to an association in series has an electrical resistance equal to the sum of the electrical resistances of the resistors and associated, consequently, this value is larger than the largest of the resistors that make up the association.
Therefore, a series combination of resistors has the following properties:

1. The electric current is the same in all resistors.
2. The ddp in association extremes is equal to the sum of the DDPS in each resistor.
3. The equivalent resistance is equal to the sum of the resistances of the resistors associated.
4. The resistor associated to present the highest electrical resistance will be subject to greater ddp.
5. The power loss is greater in higher electrical resistance resistor.
6. The total power consumed is the sum of the power consumed in each resistor.

• Association Parallel

A set of any said resistors is associated in parallel When all resistors are subjected to same potential difference.
For this to happen, All resistors must be connected to the same nodes A and B, as shown below.

To determine the equivalent resistor to an association of n resistors in parallel, we must remember that all resistors are subject to the same ddp and the total power of the association current is the sum of the electric currents in each resistor.

being:

we have:

that is:

or, Generally:

The equivalent resistor has an electrical resistance which is equal to the inverse sum of the inverses of the resistances of the resistors that make up the combination, and, consequently, equivalent resistance of the resistor is less than the smallest resistance associated.

Specific cases:

1. In the case of n resistors present the same resistance , that is, R 1 = R 2 = … = R n = R , equivalent resistor will have a given resistance:

2. If the association is composed of only two resistors R 1 and R 2 , the equivalent resistor is given by:

or

that is, the equivalent resistance is given by the product divided by the sum of the resistances of the resistors associated.

Therefore, an association in parallel It has the following properties:

1. a ddp (voltages) It is the same for all resistors;
2. total electrical current pool is the sum of the electric currents in each resistor;
3. the inverse of the equivalent resistance is equal to the sum of the inverses of the resistances associated;
4. electric current is inversely proportional to the electrical resistance, that is, most resistance becomes less electrical current;
5. Electric power is inversely proportional to electrical resistance, therefore, the largest resistor have the lower power dissipation;
6. total power consumed is the sum of the power consumed in each resistor.

• Mixed Association

We call mixed association resistors all association which can be reduced to the association in series and in parallel.

To calculate the equivalent resistor to a mixed association, we must solve the unique associations (series or parallel) that are evident and, to follow, simplify the circuit to a single unique connection.

• Calculation of Resistance Equivalent a mixed Association

Consider the association:

To solve this association, we must proceed as follows:
1. We identified and named all nodes of the association, taking care to name with the same letter that those nodes are connected by a wireless electrical resistance, they represent points that are at the same electrical potential. Thus already realized the resistors in series or parallel.

2. Launched in the same straight: the association of terminals, who will occupy the extremes, and we found, who will be among them.

3. Redesigned the resistors that straight, replacing those already in series or in parallel by their equivalent resistors, taking care to do so in terminals (letters) correct.

4. We continued this way until you reach a single resistor, which is the equivalent of the association resistor.

• Short circuit

We say that an element of a circuit is short circuit when it is subjected to a potential difference null.
Example:

In the above circuit, Lamp L 2 It is short-circuited, because it is connected to terminals A and B, presenting zero DDP due to be connected by an ideal wire. Therefore, Lamp L 2 it is off, by not passing electric current through it. The electric current, to get to point A, passes completely the ideal wire (without electrical resistance).
Under these conditions, The given circuit can be represented by the following figure.

If you have something to fix, add or any questions feel free to comment.
Coming soon post some exercises related to this topic.

Source: Physical 4 – Electrodynamics (Editora COC)